Exercises for Section 2.2

2.2.1

Consider the context-free grammar:

S -> S S + | S S * | a

1. Show how the string aa+a* can be generated by this grammar.
2. Construct a parse tree for this string.
3. What language does this grammar generate? Justify your answer.

Answer

1. S -> S S -> S S + S -> a S + S -> a a + S -> a a + a *
2. 
3. L = {Postfix expression consisting of digits, plus and multiple signs}

2.2.2

What language is generated by the following grammars? In each case justify your answer.

1. S -> 0 S 1 | 0 1
2. S -> + S S | - S S | a
3. S -> S ( S ) S | ε
4. S -> a S b S | b S a S | ε
5. S -> a | S + S | S S | S * | ( S )

Answer

1. L = {0ⁿ1ⁿ | n>=1}
2. L = {Prefix expression consisting of plus and minus signs}
3. L = {Matched brackets of arbitrary arrangement and nesting, includes ε}
4. L = {String has the same amount of a and b, includes ε}
5. L = {Regular expressions used to describe regular languages} refer to wiki

2.2.3

Which of the grammars in Exercise 2.2.2 are ambiguous?

Answer

1. No

2. No

3. Yes

   

4. Yes

   

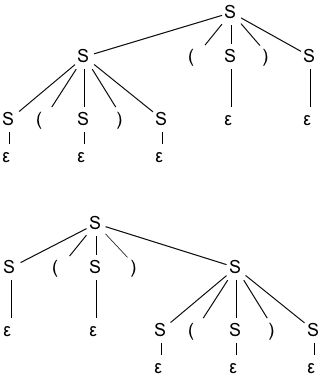
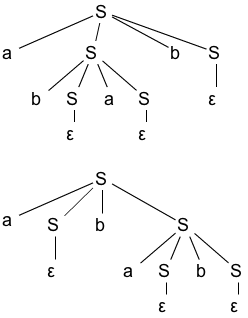
5. Yes

   

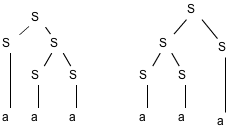
2.2.4

Construct unambiguous context-free grammars for each of the following languages. In each case show that your grammar is correct.

1. Arithmetic expressions in postfix notation.
2. Left-associative lists of identifiers separated by commas.
3. Right-associative lists of identifiers separated by commas.
4. Arithmetic expressions of integers and identifiers with the four binary operators +, -, *, /.
5. Add unary plus and minus to the arithmetic operators of 4.

Answer

1. E -> E E op | num

2. list -> list , id | id

3. list -> id , list | id

4. expr -> expr + term | expr - term | term
   term -> term * factor | term / factor | factor
   factor -> id | num | (expr)

5. expr -> expr + term | expr - term | term
   term -> term * unary | term / unary | unary
   unary -> + factor | - factor | factor
   factor - > id | num | (expr)

2.2.5

1. Show that all binary strings generated by the following grammar have values divisible by 3. Hint. Use induction on the number of nodes in a parse tree.

   num -> 11 | 1001 | num 0 | num num

2. Does the grammar generate all binary strings with values divisible by 3?

Answer

1. Proof

   Any string derived from the grammar can be considered to be a sequence consisting of 11 and 1001, where each sequence element is possibly suffixed with a 0.

   Let n be the set of positions where 11 is placed. 11 is said to be at position i if the first 1 in 11 is at position i, where i starts at 0 and grows from least significant to most significant bit.

   Let m be the equivalent set for 1001.

   The sum of any string produced by the grammar is:

   sum

   = Σ_n (2¹ + 2⁰) 2 ⁿ + Σ_m (2³ + 2⁰) 2^m

   = Σ_n 3 2 ⁿ + Σ_m 9 2^m

   This is clearly divisible by 3.

2. No. Consider the string "10101", which is divisible by 3, but cannot be derived from the grammar.

   Readers seeking a more formal proof can read about it below:

   Proof:

   Every number divisible by 3 can be written in the form 3k. We will consider k > 0 (though it would be valid to consider k to be an arbitrary integer).

   Note that every part of num(11, 1001 and 0) is divisible by 3, if the grammar could generate all the numbers divisible by 3, we can get a production for binary k from num's production:

    3k = num   -> 11 | 1001 | num 0 | num num
     k = num/3 -> 01 | 0011 | k 0   | k k
     k         -> 01 | 0011 | k 0   | k k

   It is obvious that any value of k that has more than 2 consecutive bits set to 1 can never be produced. This can be confirmed by the example given in the beginning:

   10101 is 3*7, hence, k = 7 = 111 in binary. Because 111 has more than 2 consecutive 1's in binary, the grammar will never produce 21.

2.2.6

Construct a context-free grammar for roman numerals.

Note: we just consider a subset of roman numerals which is less than 4k.

Answer

wikipedia: Roman_numerals

• via wikipedia, we can categorize the single roman numerals into 4 groups:

    I, II, III | I V | V, V I, V II, V III | I X

  then get the production:

    digit -> smallDigit | I V | V smallDigit | I X
    smallDigit -> I | II | III | ε

• and we can find a simple way to map roman to arabic numerals. For example:

  • XII => X, II => 10 + 2 => 12
  • CXCIX => C, XC, IX => 100 + 90 + 9 => 199
  • MDCCCLXXX => M, DCCC, LXXX => 1000 + 800 + 80 => 1880

• via the upper two rules, we can derive the production:

  romanNum -> thousand hundred ten digit

  thousand -> M | MM | MMM | ε

  hundred -> smallHundred | C D | D smallHundred | C M

  smallHundred -> C | CC | CCC | ε

  ten -> smallTen | X L | L smallTen | X C

  smallTen -> X | XX | XXX | ε

  digit -> smallDigit | I V | V smallDigit | I X

  smallDigit -> I | II | III | ε